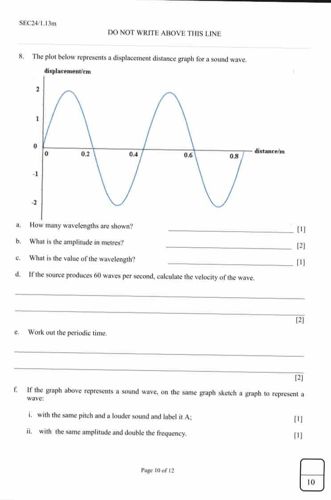
Solve the following questions based on the displacement distance graph for a sound wave: 1. How many wavelengths are shown? 2. What is the amplitude in meters? 3. What is the value of the wavelength? 4. If the source produces 60 waves per second, calculate the velocity of the wave. 5. Work out the periodic time.

Answer

1. **How many wavelengths are shown?** - From the graph, observe the peaks (crests) and through the valleys (troughs) of the wave. A full wavelength is the distance between two consecutive peaks or troughs. Counting these, we see that there are 1.5 complete wavelengths shown in the graph. 2. **What is the amplitude in meters?** - The amplitude is the maximum displacement from the rest position. From the graph, the maximum displacement reaches about 0.4 meters above the centerline and -0.4 meters below. Therefore, the amplitude is 0.4 meters. 3. **What is the value of the wavelength?** - The wavelength is the distance between two successive identical points on the wave, such as crest to crest or trough to trough. From the graph, one complete wavelength is shown to be 0.4 meters (from 0.2 to 0.6 on the distance axis). 4. **If the source produces 60 waves per second, calculate the velocity of the wave.** - The formula for wave velocity (v) is given by: \[ v = f \times \lambda \] - Where f is the frequency (60 Hz) and \( \lambda \) is the wavelength (0.4 meters): \[ v = 60 \text{ Hz} \times 0.4 \text{ m} = 24 \text{ m/s} \] - Thus, the velocity of the wave is 24 m/s. 5. **Work out the periodic time.** - The periodic time (T) is the reciprocal of the frequency. \[ T = \frac{1}{f} \] - Substituting the frequency: \[ T = \frac{1}{60} \approx 0.01667 \text{ seconds} \] - Therefore, the periodic time is approximately 0.01667 seconds.