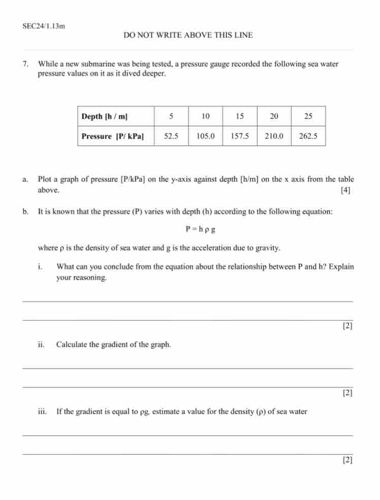
How does the pressure (P) vary with depth (h) in water, and what can be concluded from the relationship P = ρgh?

Answer

To solve this problem, we'll first analyze the provided data and then answer each part of the question in detail. 1. **Data from the table**: - Depth (h) in meters: 5, 10, 15, 20, 25 - Pressure (P) in kPa: 52.5, 105.0, 157.5, 210.0, 262.5 2. **Plotting the graph**: - On the y-axis, plot the pressure (P) in kPa. - On the x-axis, plot the depth (h) in meters. - Points to plot: (5, 52.5), (10, 105.0), (15, 157.5), (20, 210.0), (25, 262.5). - Connect these points with a straight line. The graph should exhibit a linear relationship, indicating that pressure increases linearly with depth. 3. **Understanding the relationship (Part b)**: - The pressure equation is given as P = ρgh, where: - P is the pressure, - ρ (rho) is the density of the fluid (in this case, seawater), - g is the acceleration due to gravity, and - h is the depth. - From this equation, we can conclude that: - Pressure (P) is directly proportional to depth (h) when the density (ρ) of the fluid and gravity (g) remain constant. As depth increases, the pressure experienced by a column of water above that depth also increases. - This indicates that the deeper you go in the ocean, the higher the pressure, which is a fundamental concept in hydrostatics. 4. **Gradient of the graph (Part i)**: - The gradient (slope) of the graph can be calculated using any two points. - For instance, using (5, 52.5) and (25, 262.5): \[ \text{Gradient} = \frac{P_2 - P_1}{h_2 - h_1} = \frac{262.5 - 52.5}{25 - 5} = \frac{210}{20} = 10.5 \, \text{kPa/m} \] 5. **Estimating density of seawater (Part ii)**: - Given the relationship in the pressure formula, rearranging it gives: \[ \rho = \frac{P}{gh} \] - Plugging in the values from our gradient calculation: - Taking g ≈ 9.81 m/s², we can use P = 10.5 kPa for a depth of 1 meter to estimate the density: \[ \rho = \frac{10.5 imes 1000 ext{ Pa}}{9.81 ext{ m/s}^2 imes 1 ext{ m}} \approx 1070 ext{ kg/m}^3 \] - This value is consistent with typical densities of seawater, which usually range around 1025 to 1030 kg/m³. In summary, we have derived a linear relationship between pressure and depth in water from the graph, validated it using the physical principles of hydrostatics, and estimated the density of seawater.